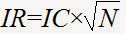by Cam Hui, Humble Student of the Markets
I have written numerous times in this space about the importance of examining your assumptions before taking any action on quantitative research.
Here is another example. I came upon a study by Alpha Architect on the tradeoff between stock selection and diversification called Diworsification: Trade-off between portfolio size and expected alpha (h/t Tadas Viskanta). The article first laid out a number of assumptions and caveats:
The results are hypothetical results and are NOT an indicator of future results and do NOT represent returns that any investor actually attained. Indexes are unmanaged, do not reflect management or trading fees, and one cannot invest directly in an index.
It went on to perform a number of simulations using Monte Carlo techniques (note key assumption in bold):
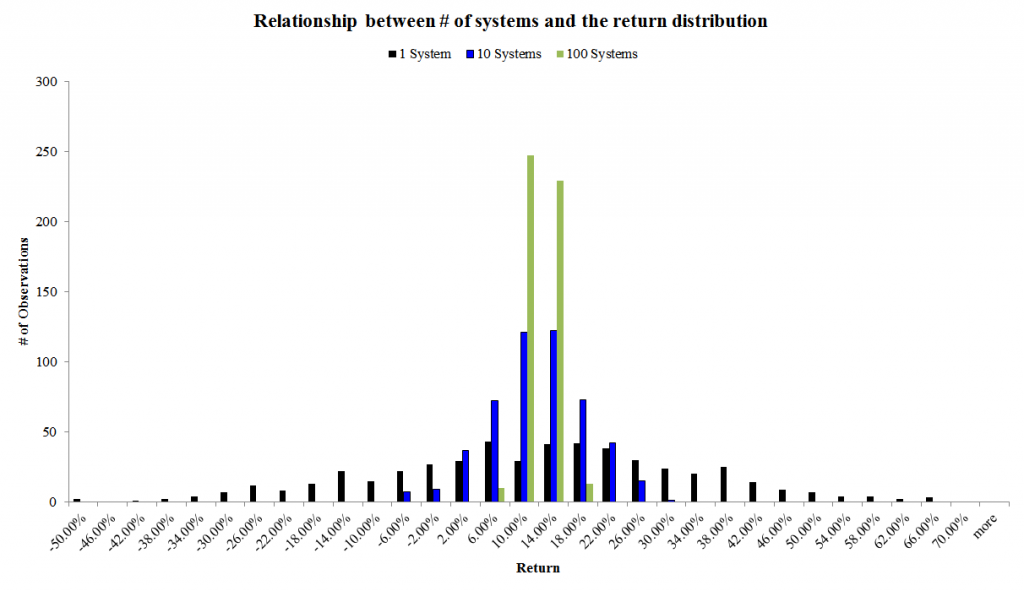
The spreadsheet conducts 1000 simulations. In each simulation run, a system can either take 1 bet, 10 bets, or 100 bets--all uncorrelated. As the chart above suggests, one can manage risk by pooling truly uncorrelated bets together. As the number of bets increases, the volatility goes to zero and the expected value becomes the observation.
In the real world stocks don't have zero correlation--correlations are much higher across equities.
Here are the conclusions based on this Monte Carlo study:
What's the bottom line?
30-50 stocks seems to be a sweet-spot where an investor eliminates portfolio idiosyncratic volatility, as additional diversification beyond this point does not help reduce volatility in any dramatic way. However, by diversifying beyond 30-50 stocks, we also prevent our portfolio from concentrating on stocks we feel are "undervalued." In other words, we probably want Warren Buffett to hold 30 or so stocks to ensure he doesn't completely blow up, but we don't want to force him to hold more than that, because it is unlikely he has more than 30 good ideas. In effect, he would be "diworsifying."
How useful is the 30-50 stock answer?
I believe that while this was a useful first step and study illustrating the tradeoffs between the effects of stock selection and diversification, I would take the conclusion of 30-50 stocks as the "sweet spot" with a grain of salt. Consider the kinds of assumptions that are made in this study:
- Individual stock returns are un-correlated
- The study does not identify the source of alpha
The second point is particularly important as it ignores Richard Grinold`s Fundamental Law of Active Management.
The Fundamental Law of Active Management
where IR = Information Ratio
IC = Information Coefficient
N = Number of independent opportunities
What Grinold means by the above formula is that a manager’s value-added (Information Ratio) is a function of his selection skill (Information Coefficient) and the number of opportunities (N) he has. In plain English, the portfolio manager should make bets in accordance with the size of his edge, or alpha.
Even then, the formula, as outlined, presents a number of implementation problems (see my previous discussion Examining your assumptions: The fundamental law of active management). They relate to time periods and optimal re-balancing; and the definition of independent opportunities (which the Alpha Architect study assumes that each stock is independent of each other).
For example, if you buy a portfolio of 100 low PE stocks, does N=1 (all low PE), 100, or somewhere in between? How different N be if it was a portfolio of 20 low PE stock?
Under these circumstances, how applicable is the Monte Carlo simulation study that 30-50 represent the "sweet spot" of diversification?
The moral of this story: Know your assumptions before coming to conclusions.
Copyright © Humble Student of the Markets