World Cup Outcomes Are Mostly Random: So Who Cares?

by Adam Butler, http://gestaltu.com

This post will be short and sweet as it’s largely an addendum to our previous post NFL Parity, Sample Size and Manager Selection.  It was motivated primarily by an interesting analysis by Tom Murphy, a physics professor at the University of California – San Diego. We greatly admire Dr. Murphy and highly recommend his blog.

Like many North Americans, Dr. Murphy doesn’t appear to be a huge fan of the beautiful game. Unlike most North Americans, his displeasure relates to an ingenious, if sterile, statistical analysis of game outcomes which concludes that soccer games are simply “well executed random events.” His article about World Cup – nay, all soccer outcomes in general – is interesting for several reasons.

First, sport outcomes and investment outcomes are dictated by different types of distributions.  In our previous calculations about the “true superiority” of an investment strategy, we used a t-distribution.  This satisfied a number of criteria we had in making the calculation, including:

1. It allowed for the full range of potential outcomes, from a 100% loss to an infinite gain;
2. It better approximated the platykurtic reality (fat tails relative to a normal distribution) of investment returns, and;
3. It provided an “accurate-enough” distribution of returns relative to our investment of time in developing the algorithm.

Of course, sports outcomes are not subject to similar characteristics as investment returns and in our previous post we used the same t-distribution for analyzing sports outcomes.  For this purpose, however, a Poisson distribution is a far more useful tool because:

1. We’re measuring scores, which are a discrete outcome of every game;
2. The average score over time is a known, measurable number;
3. Given #2, the probability that the average will be achieved is proportional to the amount of time (or games) measured, and;
4. Given #3, the probability that a score will occur as the sample size (or number of games) approaches zero is zero; there are no negative score outcomes.

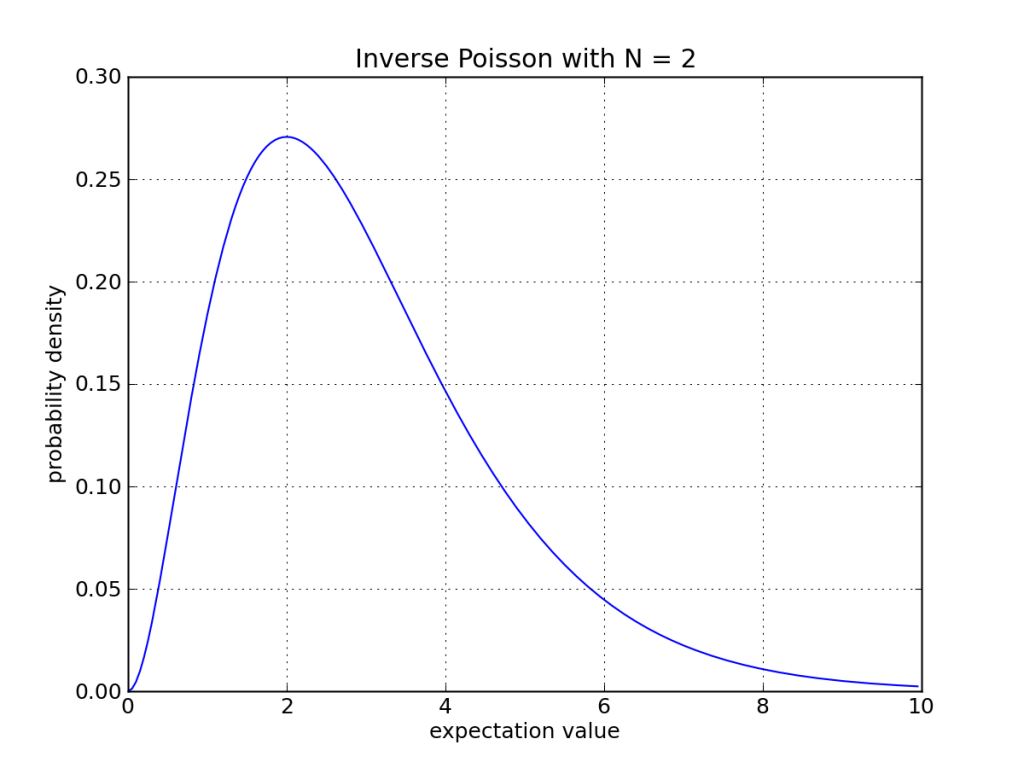
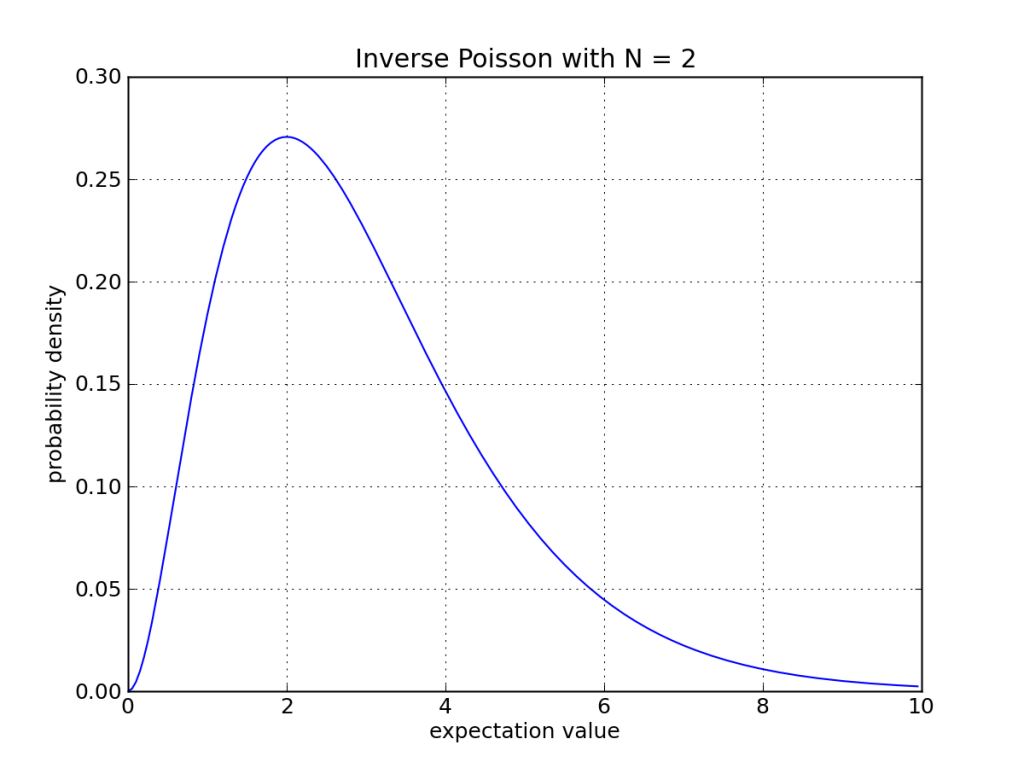
Professor Murphy, in his article, lays out a simple example:

“We can turn the Poisson distribution around, and ask: if a team scores N points, what is the probability (or more technically correct, the probability density) that the underlying expectation value is X? This is more relevant when assessing an actual game outcome.  An example appears in the plot below.  The way to read it is: if I have an expectation value of <value on the horizontal axis>, what is the probability of having 2 as an outcome? Or inversely—which is the point—if I have an outcome of 2, what is the probability (density) of this being due to an expectation value of <value on the horizontal axis>?”

The nuances of the distributions notwithstanding, our conclusions on sports and investment outcomes still stand.  Namely:

“NFL parity – and far too often, investment results – are both mirages.  Small sample sizes in any given NFL season and high levels of covariance between many investment strategies make it almost impossible to distinguish talent from luck over most investors’ investment horizons.  Marginal teams creep into the playoffs and go on crazy runs, and average investment managers have extended periods of above-average performances.”

This brings us to the second major point, which is that unlike investments where we gravitate toward risk management, in sports we tend to gravitate towards risk maximization.  In other words, to the extent that we don’t have a vested interest in the outcome, when watching a sporting event the best we can hope for is an exciting match.  In this regard, we believe the good professor has his thinking exactly right and exactly backward when, in describing why he doesn’t enjoy watching the World Cup, he says:

“…I don’t follow soccer—in part because I suspect it boils down to watching well-executed random events…What I have seen (and I have been to a World Cup game) seems to amount to a series of low-probability scoring attempts, where the reset button (control of the ball) is hit repeatedly throughout the game. I do not see a lot of long-term build-up of progress. One minute before a goal is scored, the crowd has no idea/anticipation of the impending event. American football by contrast often involves a slow march toward the goal line. Basketball has many changes of control, but scoring probability per possession is considerably higher. Baseball is a mixture: as bases load up, chances of scoring runs ticks upward, while the occasional home run pops up at random…”

Again, his characterization is spot on but his conclusion is completely wrong.  Nike seems to understand the appeal of World Cup risk with their new campaign “Risk Everything,” and the accompanying slogan “Playing it safe is the biggest risk.”

And then there’s also this video, which has over 56 million youtube hits:

It’s not in spite of the randomness, it’s because of it that the world consumes as many World Cup games as possible.  And while I’m at it, it’s why we wait with baited breath to see LeBron posterize some poor guy, why we so deeply treasure the memory of that triple play that one time, and why we recall the Immaculate Reception more clearly than just about any football play ever.

Professor Murphy pillories soccer because of it’s randomness, but we wonder if deep down, wherever he has secreted away his sense of whimsy, he would admit that his fondness for his most treasured sports memories are largely due to their incredible randomness. But we digress…

The post World Cup Outcomes Are Mostly Random: So Who Cares? appeared first on GestaltU.

Source link